a line has a slope of 4. what is the slope of any line perpendicular to this line

section 3.4 Learning Objectives

iii.4: Slope of a Line

Identify the slope of a line from the graph
Find the slope of a line given two points on a line
Find the slope and y-intercept given the equation of a line
Find the gradient of horizontal and vertical lines
Observe the slopes of parallel and perpendicular lines

Identify gradient from a graph

The mathematical definition of slope is very similar to our everyday 1. In math, gradient is used to depict the steepness and direction of lines. By but looking at the graph of a line, you lot can learn some things about its slope, especially relative to other lines graphed on the same coordinate airplane. Consider the graphs of the three lines shown below:

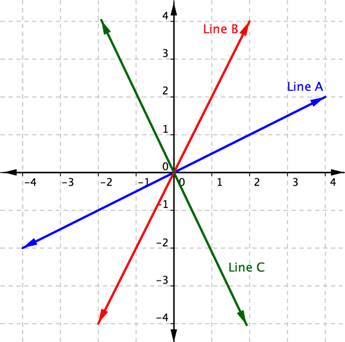
Three different lines on a graph. Line A is tilted upward. Line B is sharply titled upward. Line C is sharply tilted downward.

First, let'southward expect at lines A and B. If you imagined these lines to be hills, you would say that line B is steeper than line A. Line B has a greater slope than line A.

Next, detect that lines A and B slant upwardly as y'all move from left to correct. Nosotros say these two lines have a positive slope. Line C slants down from left to right. Line C has a negative slope. Using 2 of the points on the line, you can find the gradient of the line past finding the rise and the run. The vertical change betwixt two points is called the rise, and the horizontal change is called the run. The gradient equals the ascent divided by the run: [latex] \displaystyle \text{Gradient }=\frac{\text{ascent}}{\text{run}}[/latex].

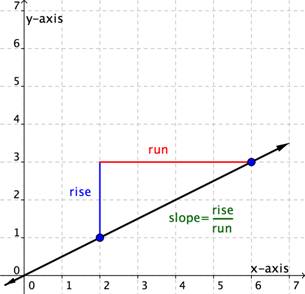
A line that crosses the points (2,1) and (6,3). A blue line labeled Rise goes up two units from the point (2,1). A red line labeled Run goes left from the point (6,3) so that it forms a triangle with the main line and the Rise line. A formula says slope equals rise over run.

You tin determine the slope of a line from its graph by looking at the rise and run. One characteristic of a line is that its slope is constant all the fashion forth information technology. Then, y'all tin can choose any 2 points forth the graph of the line to figure out the slope. Let'south look at an example.

Instance 1

Use the graph to find the gradient of the line.

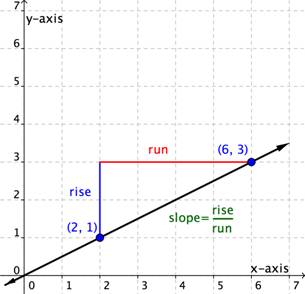
This line will have a slope of [latex] \displaystyle \frac{i}{2}[/latex] no matter which two points you pick on the line. Try measuring the slope from the origin, [latex](0,0)[/latex], to the point [latex](6,3)[/latex]. You will find that the [latex]\text{rise}=3[/latex] and the [latex]\text{run}=six[/latex]. The slope is [latex] \displaystyle \frac{\text{rise}}{\text{run}}=\frac{3}{vi}=\frac{i}{ii}[/latex]. It is the same!

Permit's await at some other example.

Example 2

Use the graph to find the slope of the two lines.

When y'all look at the 2 lines, y'all tin can see that the blueish line is steeper than the carmine line. It makes sense the value of the gradient of the blue line, 4, is greater than the value of the gradient of the ruby line, [latex] \displaystyle \frac{one}{4}[/latex]. The greater the slope, the steeper the line.

Distinguish betwixt graphs of lines with negative and positive slopes

Direction is important when it comes to determining slope. Information technology's important to pay attending to whether yous are moving up, downwards, left, or right; that is, if you are moving in a positive or negative direction. If yous go up to go to your second bespeak, the rise is positive. If y'all go down to get to your second betoken, the rising is negative. If you lot get right to get to your second bespeak, the run is positive. If yous become left to become to your 2d point, the run is negative.

In the post-obit two examples, you volition see a slope that is positive and one that is negative.

Example 3 (Avant-garde)

Find the slope of the line graphed below.

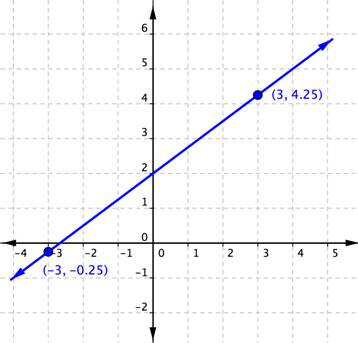
The adjacent instance shows a line with a negative gradient.

Example 4

Find the slope of the line graphed below.

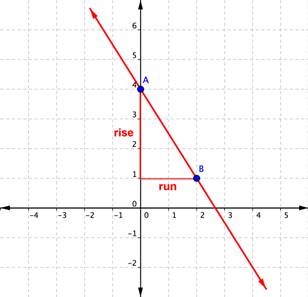
A downward-sloping line that goes through points A and B. Point A is (0,4) and point B is (2,1). The rise goes down three units, and the run goes right 2 units.

In the instance above, you could take also plant the slope by starting at bespeak B, ascension [latex]+3[/latex] (up) and and then running [latex]-2[/latex] (left) to get in at indicate A. The upshot is all the same a slope of [latex]\displaystyle\frac{\text{rise}}{\text{run}}=\frac{+iii}{-2}=-\frac{3}{2}[/latex].

Find the slope given two points on the line

Y'all've seen that y'all tin can find the slope of a line on a graph by measuring the rise and the run. You can also observe the gradient of a line without its graph if you lot know the coordinates of any two points on that line. Every betoken has a ready of coordinates: an x-value and a y-value, written as an ordered pair (x, y). The x-value tells you where a point is horizontally. The y-value tells you where the point is vertically.

Consider ii points on a line—Point one and Point 2. Bespeak 1 has coordinates [latex]\left(x_{ane},y_{1}\right)[/latex] and Point 2 has coordinates [latex]\left(x_{2},y_{two}\right)[/latex].

A line with its rise and run. The first point on the line is labeled Point 1, or (x1, y1). The second point on the line is labeled Point 2, or (x2,y2). The rise is (y2 minus y1). The run is (x2 minus X1).

The rise is the vertical change between the 2 points, which is the difference between their y-coordinates. That makes the ascension [latex]\left(y_{ii}-y_{1}\right)[/latex]. The run between these two points is the difference in the 10-coordinates, or [latex]\left(x_{2}-x_{1}\right)[/latex].

So, [latex] \displaystyle \text{Slope}=\frac{\text{rise}}{\text{run}}[/latex] or [latex] \displaystyle one thousand=\frac{{{y}_{2}}-{{y}_{1}}}{{{x}_{2}}-{{x}_{one}}}[/latex]

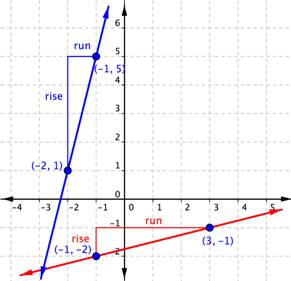
In the case below, you'll run across that the line has two points each indicated equally an ordered pair. The point [latex](0,2)[/latex] is indicated as Indicate 1, and [latex](−2,6)[/latex] as Bespeak 2. Then you are going to movement from Point 1 to Betoken 2. A triangle is drawn in higher up the line to help illustrate the rise and run.

A line going through Point 1, or (0,2), and Point 2, or (-2,6). The rise is 4 and the run is -2.

You lot can see from the graph that the rising going from Point 1 to Bespeak 2 is iv, because you lot are moving iv units in a positive direction (up). The run is [latex]−2[/latex], because you are and so moving in a negative direction (left) 2 units. Using the slope formula,

[latex] \displaystyle \text{Slope}=\frac{\text{rise}}{\text{run}}=\frac{iv}{-2}=-2[/latex].

You do not demand the graph to discover the gradient. Y'all can just use the coordinates, keeping careful track of which is Point 1 and which is Point two. Let's organize the information most the two points:

Proper name	Ordered Pair	Coordinates
Point 1	[latex](0,2)[/latex]	[latex]\begin{array}{l}x_{1}=0\\y_{ane}=2\end{array}[/latex]
Betoken 2	[latex](−ii,half-dozen)[/latex]	[latex]\begin{array}{fifty}x_{2}=-ii\\y_{2}=six\terminate{array}[/latex]

The slope, [latex]m=\frac{y_{2}-y_{1}}{x_{two}-x_{1}}=\frac{6-2}{-ii-0}=\frac{4}{-2}=-2[/latex]. The slope of the line, m, is [latex]−ii[/latex].

It doesn't thing which point is designated as Point 1 and which is Point 2. Y'all could have called [latex](−2,half-dozen)[/latex] Signal i, and [latex](0,2)[/latex] Point ii. In that instance, putting the coordinates into the slope formula produces the equation [latex]m=\frac{2-vi}{0-\left(-2\right)}=\frac{-iv}{2}=-2[/latex]. Once more, the slope is [latex]m=-two[/latex]. That's the same gradient every bit before. The important thing is to exist consistent when you subtract: you must always decrease in the aforementioned order.

Instance 5

What is the gradient of the line that contains the points [latex](5,v)[/latex] and [latex](four,2)[/latex]?

The case below shows the solution when yous contrary the lodge of the points, calling [latex](5,5)[/latex] Point 1 and [latex](iv,2)[/latex] Point 2.

Case 6

What is the slope of the line that contains the points [latex](5,5)[/latex] and [latex](iv,2)[/latex]?

Notice that regardless of which ordered pair is named Point 1 and which is named Indicate ii, the slope is however three.

Case 7 (Advanced)

What is the slope of the line that contains the points [latex](3,-6.25)[/latex] and [latex](-1,8.5)[/latex]?

Detect the gradient and y-intercept given the equation of the line:

Nosotros can also determine the slope of a line from the equation of the line. To exercise this, we first need to write the equation in Slope-Intercept Form.

Gradient-Intercept Form: [latex]y=mx+b[/latex]

To write an equation in Slope-Intercept Form, nosotros need to solve for [latex]y[/latex] in the equation. In one case the equation is in this grade, nosotros know a couple of things simply by looking at the equation.

The coefficient of [latex]x[/latex] is the slope of the line.

The y-intercept is the signal [latex](0,b)[/latex].

Example 8

Detect the gradient and y-intercept of the line with equation [latex]y=-3x+seven[/latex]

Example 9

Notice the gradient and y-intercept of the line with equation [latex]-4x+2y=-6[/latex]

Instance 10

Find the slope and y-intercept of the line with equation [latex]y=\frac{three}{5}ten[/latex]:

Find the slope of horizontal and vertical lines

So far you've considered lines that run "uphill" or "downhill." Their slopes may be steep or gradual, but they are always positive or negative numbers. Only there are two other kinds of lines, horizontal and vertical. What is the gradient of a flat line or level ground? Of a wall or a vertical line?

The line y=3 crosses through the point (-3,3); the point (0,3); the point (2,3); and the point (5,3).

Let's consider a horizontal line on a graph. No affair which two points y'all cull on the line, they will e'er take the aforementioned y-coordinate. The equation for this line is [latex]y=iii[/latex]. The equation can too exist written as [latex]y=\left(0\right)x+iii[/latex].

Using the grade [latex]y=0x+3[/latex], you tin can come across that the slope is 0. You can as well use the gradient formula with two points on this horizontal line to calculate the slope of this horizontal line. Using [latex](−3,3)[/latex] as Signal i and (2, 3) as Point two, you get:

[latex] \displaystyle \begin{assortment}{l}m=\frac{{{y}_{2}}-{{y}_{i}}}{{{x}_{2}}-{{x}_{ane}}}\\\\m=\frac{iii-3}{2-\left(-3\right)}=\frac{0}{v}=0\end{assortment}[/latex]

The gradient of this horizontal line is 0.

Let'southward consider whatsoever horizontal line. No thing which two points yous choose on the line, they will always have the same y-coordinate. So, when y'all employ the slope formula, the numerator will always be 0. Zero divided by any not-zero number is 0, so the slope of any horizontal line is ever 0.

The equation for the horizontal line [latex]y=3[/latex] is telling you lot that no matter which two points you choose on this line, the y-coordinate will ever be 3.

How nearly vertical lines? In this case, no affair which two points you cull, they will always have the same x-coordinate. The equation for the line shown below is [latex]x=2[/latex].

The line x=2 runs through the point (2,-2), the point (2,1), the point (2,3), and the point (2,4).

There is no fashion that this equation tin exist put in the slope-bespeak form, as the coefficient of y is [latex]0[/latex].

So, what happens when you use the slope formula with two points on this vertical line to summate the slope? Using [latex](2,i)[/latex] as Betoken one and [latex](2,iii)[/latex] every bit Point ii, you get:

[latex] \displaystyle \begin{array}{50}chiliad=\frac{{{y}_{2}}-{{y}_{1}}}{{{ten}_{two}}-{{x}_{1}}}\\\\yard=\frac{3-i}{2-2}=\frac{2}{0}\stop{array}[/latex]

But division by nothing is undefined for the set of real numbers. Because of this fact, it is said that the slope of this vertical line is undefined. This is truthful for all vertical lines—they all have a slope that is undefined.

Example 11

What is the slope of the line that contains the points [latex](3,2)[/latex] and [latex](−eight,two)[/latex]?

Example 12

Detect the slope and y-intercept of the line with equation [latex]y=-2[/latex] :

Instance thirteen

Notice the gradient and y-intercept of the line with equation [latex]x=3[/latex]:

Characterize the slopes of parallel and perpendicular lines

When y'all graph two or more linear equations in a coordinate plane, they more often than not cantankerous at a bespeak. Notwithstanding, when two lines in a coordinate plane never cantankerous, they are called parallel lines. You volition also await at the case where two lines in a coordinate aeroplane cantankerous at a right angle. These are called perpendicular lines. The slopes of the graphs in each of these cases have a special relationship to each other.

Parallel lines are two or more lines in a plane that never intersect. Examples of parallel lines are all around us, such equally the contrary sides of a rectangular flick frame and the shelves of a bookcase.

Line y=2x+3 and line y=2x-3. Caption says Equations of parallel lines will have the same slopes and different intercepts.

Perpendicular lines are two or more lines that intersect at a 90-degree angle, like the two lines fatigued on this graph. These 90-degree angles are too known as correct angles.

Two lines that cross to form a 90 degree angle.

Perpendicular lines are also everywhere, not simply on graph newspaper but too in the world around the states, from the crossing pattern of roads at an intersection to the colored lines of a plaid shirt.

Parallel Lines

Two not-vertical lines in a plane are parallel if they have both:

the same slope
different y-intercepts

Any two vertical lines in a plane are parallel.

Find the slopes of parallel lines

Example 14

Find the gradient of a line parallel to the line [latex]y=−3x+4[/latex].

Example 15

Make up one's mind whether the lines [latex]y=6x+5[/latex] and [latex]y=6x–1[/latex] are parallel.

Find the slopes of perpendicular lines

Perpendicular Lines

Two non-vertical lines are perpendicular if the slope of 1 is the negative reciprocal of the slope of the other. If the slope of the first equation is [latex]\frac{2}{3}[/latex], then the slope of the 2d equation will need to be [latex]-\frac{3}{two}[/latex] for the lines to be perpendicular.

You can also check the two slopes to see if the lines are perpendicular by multiplying the two slopes together. If they are perpendicular, the product of the slopes will be [latex]−1[/latex]. For instance, [latex] \frac{2}{3}\cdot-\frac{three}{two}=-1[/latex].

Example 16

Find the gradient of a line perpendicular to the line [latex]y=2x–6[/latex].

To find the gradient of a perpendicular line, find the reciprocal, [latex] \displaystyle \frac{two}{1}[/latex], which is [latex]\frac{1}{ii}[/latex],and and then discover the opposite of this reciprocal, which is [latex] \displaystyle -\tfrac{1}{2}[/latex].

Annotation that the product [latex]2\left(-\frac{1}{2}\right)=\frac{2}{1}\left(-\frac{1}{2}\correct)=-1[/latex], and then this means the lines are perpendicular.

In the case where one of the lines is vertical, the slope of that line is undefined and it is not possible to summate the product with an undefined number. When one line is vertical, the line perpendicular to it will be horizontal, having a slope of zilch ([latex]thou=0[/latex]).

Example 17

Determine whether the lines [latex]y=−8x+5[/latex] and [latex] \displaystyle y\,\text{=}\,\,\frac{ane}{viii}10-1[/latex] are parallel, perpendicular, or neither.

In this last example, you volition piece of work with an equation of a line given in Standard Class instead of Slope-Intercept Form as the previous examples have been given in.

Example 18

Consider the equation: [latex]8x-5y=x[/latex]

What is the slope of a line that is parallel to the in a higher place line?

What is the slope of a line that is perpendicular to the above line?

Summary

Slope describes the steepness of a line. The slope of whatsoever line remains abiding forth the line. The slope tin can also tell you information about the management of the line on the coordinate plane. Gradient tin can be calculated either by looking at the graph of a line or by using the coordinates of whatever 2 points on a line. At that place are two common formulas for slope: [latex] \displaystyle \text{Gradient }=\frac{\text{rise}}{\text{run}}[/latex] and [latex] \displaystyle m=\frac{{{y}_{2}}-{{y}_{1}}}{{{ten}_{2}}-{{10}_{1}}}[/latex] where [latex]m=\text{slope}[/latex] and [latex] \displaystyle ({{10}_{1}},{{y}_{1}})[/latex] and [latex] \displaystyle ({{x}_{2}},{{y}_{ii}})[/latex] are two points on the line.

The images below summarize the slopes of unlike types of lines.

Uphill line with positive slope has a line that starts at the bottom-left and goes into the top-right of the graph. Downhill line with negative slope starts in the top-left and ends in the bottom-right part of the graph. Horizontal lines have a slope of 0. Vertical lines have an undefined slope.

yuegary2001.blogspot.com

Source: https://courses.lumenlearning.com/slcc-elementaryalgebra/chapter/3-4-slope-of-a-line/